Pascal's Triangle

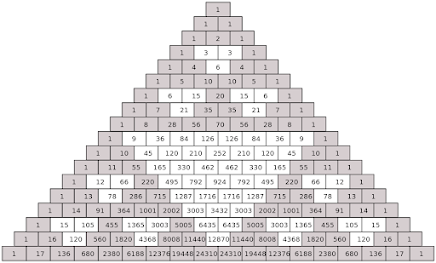
The pascals triangle is a truly scintillating arrangement of numbers that gives us the coefficients of a binomial expression when expanded. Pascal’s Triangle was named after 17th century french mathematician, Blake Pascal. It uses a special sequence commonly known as the Fibonacci Sequence. The  Fibonacci Sequence involves a series of numbers in which each number is the sum of the two numbers before. The sum goes in the next tier. 

To build the triangle, it always must start with a 1. Imagine a 0 on either side of the 1. Now add 1 to each of the 0’s. You will get 1, 1 right? The two numbers would be the sequence of the second row. Then, imagine 0 on opposite sides again. Add together the numbers beside each other and write the sum on the third row. This can go on, and on until...well...forever. 

Now, you can figure out a binomial expression as it gives the coefficients. Lets start of with (a+b)^0 which is obviously 1 as anything to the power of 0 is 1. The coefficient is 1 and therefore, is the first number of the triangle. Then, (a+b)^1 = a+b. The coefficients of them are both 1, so there are two 1’s, which is evident by the second layer. The same goes with (a+b)^2...(a+b)^3 and so on. This method was designed to make solving binomial expressions much easier from, (x+y)^0 to (x+y)^n. 

However, the Pascal’s triangle isn’t only used for binomial expressions -- it can be used to find probability. For instance, the probability of a couple having 2 girls and 3 boys. Using this sequence, there would be a probablity of 31.25%. I stumbled accross this topic during a Russian Math lesson, and I was engrossed in this math masterpiece. When expanding higher powers like 3 and 4, it was very difficult to memorise the order. This sequence made it much easier to comprehend and memorise. 

Overall, the pascal’s triangle is a beautiful and incredible assortment of numbers. It revolutionized how we know math and helped binomials become much easier to handle. Scientists still haven't uncovered all the potential this triangle holds. Recent research has also discovered a way to expand it to different kinds of polynomials. This triangle holds secrets that are yet to uncover...well why don’t you take a look at it for yourself?